In Remembrance of Tom Wieting (1938-2021)
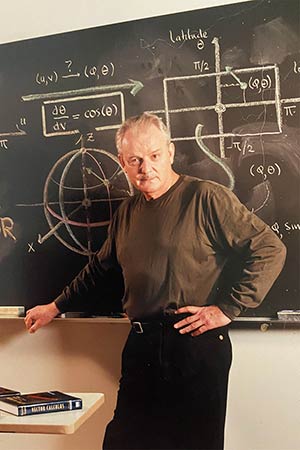
from Ray Mayer
from Nicholas Wheeler
from David Griffiths
Obituary in The Oregonian
Travels with Tom slideshow
Tom reciting Poe's The Raven
Capturing Infinity: The Circle Limit Series of M.C. Escher, by Tom Wieting
Tom had broad interests in analysis in general, and in mathematical physics. I think he talked with Nick Wheeler and David Griffiths about physics more than he talked to anyone in the math department about math, and they know more about his physics interests than I do. He usually wanted to start with axioms (even when doing physics), and he was interested in formal logic. He once told me that he introduced the Zermelo Fraenkel Axioms in his beginning calculus class because he couldn't talk about sets without knowing what their properties were. I don't know whether he was serious, or whether he just wanted to see my reaction, but he did write a set of notes called "Mathematics 000", which started with the Zermelo Fraenkel Axioms and developed the real numbers. He was also interested in low dimensional geometry e.g. plane ornaments, tilings, crystals, maps. He was interested in Esher's drawings, and once gave a lecture at the Portland Art Museum about Esher's drawings. He published a book on crystallographic groups. He once began a book on dynamical systems (at a time when all books on dynamical systems were research monographs), and I believe he had a contract with a publisher to produce a draft by a certain date, but the background materials kept growing (he had to cover all of the background on measure theory, probably starting with ZF). The draft deadline passed, and the number of volumes grew, and books on dynamical systems by other authors appeared, and after quite a few years the project died.
Tom was scheduled to give a Reed math colloquium talk on Godel's theorem when he got his cancer diagnosis and cancelled the talk. He was very excited about his proposed talk, and said the topic was something every mathematics undergraduate should be familiar with. During the time was getting cancer therapy he mentioned the talk, and he was planning to give it after he recovered. I would like to have heard the talk.
Tom's lectures were very polished. When he spoke, it sounded like every sentence had been carefully rehearsed. When I looked at the chalkboard at the end of a talk. I had the feeling that he had planned the position of every symbol on the board before he started the talk. He had strong feelings about the "right way" to do things. For example, the usual way of denoting a color is by a triple [r,g,b] where r,g,b, are numbers in the interval [0,1] denoting the levels of red, green and blue respectively. Tom used the notation [r,b,g], which he thought was more natural.
Ray Mayer
Professor of Mathematics, Emeritus
It was on a warm September afternoon in 1965 that I abandoned my basement office, hiked up to the 3rd floor of Eliot Hall to introduce myself to our new mathematician.
I was surprised to be asked, near the end of that initial conversation, what physicists made of the fact that the left side of the equation fundamental to their field (Newton’s F = mẍ) transforms covariantly, while the ẍ on the right transforms contravariantly, with the consequence that—except with respect to a very restricted class of transformations—the equation falls apart when transformed. The question (resolved by my observation that the mass factor transforms as a covariant tensor of second rank) struck me as exceptionally perceptive, since it touches on a point seldom addressed in introductory physics texts. I came away from that meeting pleased to have discovered that Tom was “my kind of mathematician.”
Thus began the conversation that Tom and I continued for well more than fifty years... often, in years long gone, in after-lunch strolls through the Rhododendron Garden and hikes in the Canyon.
It was evident from the beginning that Tom was a mathematician apart from most other mathematicians, one who—in continuation of an ancient tradition—drew his inspitation from deep curiosity about the way the world works, at the most fundamental level. He reveled in the mysterious fact that mathematics is the “language of Nature.” Tom was what in another age was called a “natural philosopher,” in the sense understood by the giants he most admired (Galileo, Descartes, Newton, Leibniz, Huygens, successive generations of Bernoulis, Euler, Gauss), before natural philosophy resolved into physics, mathematics and their ever more finely partitioned sub-fields.
Tom was a broadly informed mathematical generalist, most attracted to subject areas that (unlike, say, number theory) possessed a discernable over-all architecture. He was seldom tempted to drill down into the details of specific calculations because such details are problem-specific, and tend to obscure the view of grand designs. In each of the many brief essays that can be found on his website his creative effort was to capture the uncompromisingly abstract structural essentials of the point at issue. Though deeply informed about the history of mathematics, and boundless in his admiration for its creative giants, he resisted any temptation to insert allusions to historical or biographical circumstance, or even to provide motivational of summary remarks that would—however helpful to the reader—detract from the crystaline abstract purity of his arguments. He wrote never for publication (in a style that most editors would find unacceptable; he and I shared this self-indulgent reticence—tacitly sanctioned in those days by the college—for distinct reasons that we never discussed) but for the edification of an idealized reader (God?), material that for the rest of us is often not easy to understand, at least on a first reading.
His stance when he wrote about physics—most commonly about the foundations of quantum mechanics and general relativity—was similar. That work was informed never by an appeal to physical intuition, invariably by his cultivated sense of mathematical nicety, was sometimes unconvincingly eccentric and lies beyond the easy reach of most physicists.
Tom was, in short, an aesthetician, as sensitive to the manifest beauty of the Nature in which he loved to hike and float as he was to the hidden beauty of the ideas that have been—over a span of several millennia—devised to render it comprehensible. He lectured repeatedly, to diverse audiences, about Descartes’ theory of the rainbow, and travelled to the Alhambra to view the 14th Century Moorish realization of the seventeen possible “wallpaper groups,” the sight of which in 1922 had inspired the young M. C. Escher (one of Tom’s favorite graphic artists) and to the mathematical theory of which Tom devoted his only book: Mathematical Theory of Chromatic Plane Ornaments (1982).
Tom’s lectures and seminar presentations were themselves celebrtated works of art: he spoke invariably without notes, for precisely the allotted time, and his meticulously rendered graphics filled but never exceeded the available blackboard space. His physics seminars—for many years eagerly anticipated annual events—were often devoted to clarification of puzzling, paradoxical, seemingly-impossible results of mathematical or physical importance. Delivered in his well-modulated large voice, replete with the contextual remarks so notably absent from his essays, they were invariably interesting, informative, the very model of comprehensible lucidity. Tom drew his source material more often from monographs than from papers, and when he lectured had invariably on display a short stack of books, as though to lend (superfluous) authenticity to his remarks, as though they were friends who deserved to participate in the event.
Nicholas Wheeler
A. A. Knowlton Professor of Physics, Emeritus
15 October 2021
Tom was a genuine Renaissance man, interested in everything, from Islamic art to Gödel’s theorem. He was, as far as I know, the only person in the Division to teach in Hum 110, and he offered many courses in the MALS program. He gave a famous lecture on the rainbow, a surprisingly complex and subtle optical phenomenon that he managed to make intelligible to audiences of physicists and laymen alike. He was a wonderful speaker, whose eloquence was legendary and blackboardsmanship was exquisite. Among Reed’s mathematicians he was the most knowledgable about physics, and almost always the math advisor on math/physics theses. In a department dominated (as it seemed to us) by algebraists and geometers, he specialized in analysis (though he taught everything from linear algebra to mathematical logic), and he always argued in favor of practical, usable mathematics (which was amusing to us in the physics department, because from our perspective he approached every problem from the most abstract and esoteric angle). Tom was a voracious reader, and knew many of the classic papers in mathematical physics much better than we did. I learned more from him about the behavior of self-adjoint operators in Hilbert space than I ever did in graduate courses on quantum field theory. He wrote elegant treatises on a wide variety of subjects, which he shared with a few friends (though, as far as I know, he never published them). Like any good mathematician he disdained to tell the reader why he was doing what he was doing—what question he was trying to answer, or what problem he was trying to solve. You were supposed to figure that out for yourself. After we all retired Tom, Nick Wheeler, and I would often repair to the Woodstock Wine and Deli for a leisurely lunch and discuss his worries about the insecure foundations of elementary particle physics, or his doubts about the validity of Bell’s theorem, or his obsession with source-free electromagnetic fields (which I only recently came to understand was inspired by the Gnostic notion of a perfect universe composed entirely of light). He was a gentleman and a scholar, and a cherished friend.
David Griffiths
Professor of Physics, Emeritus
September 30, 2021